Numerical simulation of temperature distribution during radio frequency tempering of pollack surimi based on dielectric properties
-
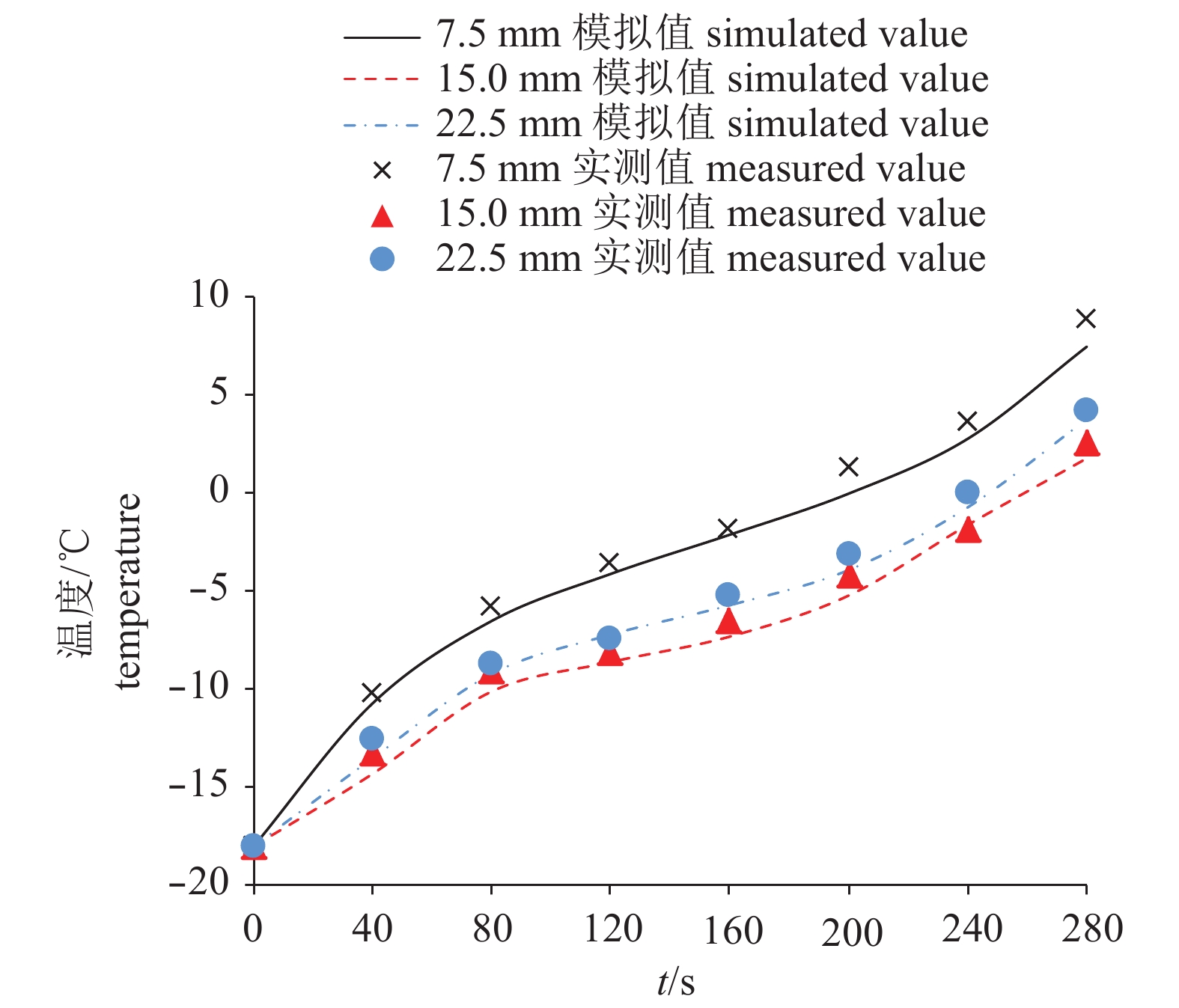
摘要: 无线电波加热具有选择加热的特性，随着被解冻物料温度的升高，物料的介电特性随温度的变化会导致热点部位吸收能量多，升温迅速，而相应的较冷部位吸收能量少，致使温度分布差异增大，物料品质大幅下降。提高加热均匀性是无线电波解冻中被最为关注的问题。文章以阿拉斯加狭鳕(Theragra chalcogramma)鱼糜为研究对象，采用同轴探针方法测定了狭鳕鱼糜的介电特性，得出了狭鳕鱼糜介电常数和介电损失随温度变化的回归方程；在此基础上应用COMSOL Multiphysics软件，对无线电波解冻狭鳕鱼糜的物理过程进行动态数值模拟，以有限元方法数值求解电磁波耗散及热传递耦合方程并通过实验测定对温度计算值进行验证。结果表明，基于介电特性的耦合方程可准确地模拟无线电波加热过程的温度变化，从而用于预测物料的温度分布。Abstract: Radio frequency tempering has properties of selective heating. As the increase of temperature, the hotter part of the thawed material absorbs more energy; heat increases rapidly with the change of dielectric properties, while the corresponding cooler part absorbs less energy, which leads to the difference of temperature distribution and will reduce the quality of thawed materials. Increasing uniformity of temperature distribution is most concerned during radio frequency. In this paper, the dielectric properties of pollock (Theragra chalcogramma) surimi were determined by open ended coaxial-line probe method. The regression equations of the dielectric constant and dielectric loss were obtained. Based on the dielectric property, a numerical simulation of the temperature distribution during radio frequency tempering of pollack surimi was carried out by COMSOL Multiphysics software. In order to obtain the physical process of radio frequency tempering for dynamic numerical simulation, the finite element method was used to solve the electromagnetic wave dissipation and heat transfer coupling equation, and was experimentally verified by conducting radio frequency tempering on pollack surimi. The results show that the simulation of coupling equation based on dielcetric property can predict the temperature distribution of the samples accurately during radio frequency tempering.
-
Keywords:
- pollock surimi /
- dielectric properties /
- radio frequency /
- tempering /
- numerical simulation
-
巢湖地处长江中下游的安徽省中部，是我国著名的五大淡水湖之一，其地理坐标为东径117°16′54″~117°51′46″，北纬30°25′28″~31°43′28″，属长江左岸水系。全流域面积9 173 km2，涉及7县2市，地貌总体呈南北高，中间低，杭埠河、白石天河、兆河等主要河流呈向心状分布。湖区跨越2市、3县、1区，是沿湖地区工农业生产和群众生活用水的重要水源，是渔业生产的重要基地。农业部渔业生态环境监测中心将其作为编制2004年《中国渔业生态环境状况公报》的重点渔业水域，进行渔业生态环境监测。本文通过对调查、测定的结果进行综合分析，应用层次分析决策法对巢湖渔业生态环境进行评价。
1. 采样点选择及监测项目
通过对近年巢湖渔业调查分析，结合水域特点及入湖河流状况，确定了5月份巢湖鱼类生态环境监测点，见图 1。
监测项目：
(1) 生物环境监测：叶绿素a、浮游植物、浮游动物；
(2) 水质监测：水温、透明度、pH、溶解氧、电导率、总氮、总磷、非离子氨、高锰酸盐指数、铜、锌、铅、镉、汞、砷、石油类、挥发酚。
2. 层次分析
人们在决策过程中，往往会遇到无法定量化的因素，因而影响到决策的质量。美国著名运筹学家Saaty教授于20世纪70年代中期创立的层次分析法(Analytic Hierarchy Process，AHP)是一种能用来处理复杂的社会、政治、经济、科学技术等决策问题的新方法[1, 2]。渔业生态环境综合评价涉及到水域的生物环境和水质环境等多个因素，其环境的优劣类似一个多目标决策问题。它可在确定渔业最优环境这个总目标下，划分各分指标层和方案层，结合水环境综合评价的特点，建立评定渔业最优环境的层次结构模型。
2.1 建立递阶层次结构[3, 4, 9]
根据巢湖水域渔业环境的特点，结合生物监测、水质监测的结果，建立了巢湖水域渔业环境优劣评价系统的递阶层次结构。见图 2。
图 2中，最上层是目标层，各监测点渔业环境的优劣排序；第二层是指标层，该层因素为渔业环境两大类环境因子，因两大类环境因子优劣判断标准有差异，故这里把它们区分开，再细分成分指标层；最低层为方案层，即由不同指标数值组成的各监测点。
2.2 构造各层因素间的比较判断矩阵[5-8]
2.2.1 建立三标度比较矩阵
根据图 2的递阶层次结构，建立最上层至最低层逐层构造有关因素之间的两两比较判断矩阵。亦即对上一层某因素而言，在其下一层次上所有与它关联的因素中依次两两比较两者的重要性或有利关系，对指标而言按“重要”、“同等重要”和“不重要”，对方案而言按“有利”、“同样有利”和“不利”，分别用“2”、“1”和“0”三种数值标度定量表示。由此得出的三标度矩阵，它表示了各因素之间相对于上一层某因素的重要性或有利关系。如A因素与下一层次中的B1，B2，…，Bn有联系，其三标度矩阵的一般形式为：
A B1 B2 … Bn B1 b11 b12 … b1n B2 b21 b22 … b2n $\vdots $ $\vdots $ $\vdots $ $\vdots $ $\vdots $ Bn bn1 bn2 … bnn 或$ B=\left[\begin{array}{cccc} b_{11} & b_{12} & \cdots & b_{1 n} \\ b_{21} & b_{22} & \cdots & b_{2 n} \\ \vdots & \vdots & \vdots & \vdots \\ b_{n 1} & b_{n 2} & \cdots & b_{n n} \end{array}\right]=\left(b_{i j}\right)_{n \times n}$其中
$$ b_{i j}= \begin{cases}2 & \text { 在 } A \text { 因素下, } B_i \text { 比 } B_j \text { 重要或有利 } \\ 1 & \text { 在 } A \text { 因素下, } B_i \text { 与 } B_j \text { 同样重要或有利 } \\ 0 & \text { 在 } A \text { 因素下, } B_i \text { 没有 } B_j \text { 重要或有利 }\end{cases} $$ 且有bii=1，即在A因素下，Bi自身比较，重要性或有利关系相同。
按上述方法，巢湖宜渔指标，据专家定权法建立三标度比较矩阵分别为：
A1—B A1 B1 B2 B3 B4 B5 B6 B7 ri 基点base point B1 1 2 0 0 2 2 0 7 rmax=13→B3
rmin=1 →B2
dm(B3: B2)=7B2 0 1 0 0 0 0 0 1 B3 2 2 1 2 2 2 2 13 B4 2 2 0 1 2 2 2 11 B5 0 2 0 0 1 0 0 3 B6 0 2 0 0 2 1 0 5 B7 2 2 0 0 2 2 1 9 B1—C B1 C1 C2 C3 C4 C5 ri 基点base point C1 1 0 2 2 1 6 rmax=9→C2
rmin=1 →C3
dm(C2: C3)=5C2 2 1 2 2 2 9 C3 0 0 1 1 0 1 C4 0 0 2 2 0 3 C5 1 0 2 2 1 6 B2—C B2 C1 C2 C3 C4 C5 ri 基点base point C1 1 2 2 0 0 5 rmax=9→C5
rmin=1→C3
dm(C5: C3)=3C2 0 1 2 0 0 3 C3 0 0 1 0 0 1 C4 2 2 2 1 0 7 C5 2 2 2 2 1 9 B3—C B3 C1 C2 C3 C4 C5 ri 基点base point C1 1 2 2 2 2 9 rmax=9→C1
rmin=1 →C4
dm(C1: C4)=5C2 0 1 0 2 0 3 C3 0 2 1 2 0 5 C4 0 0 0 1 0 1 C5 0 2 2 2 1 7 B4—C B4 C1 C2 C3 C4 C5 ri 基点base point C1 1 0 0 0 0 1 rmax=9→C5
rmin=1→C1
dm(C5: C1)=3C2 2 1 2 2 0 7 C3 2 0 1 2 0 5 C4 2 0 0 1 0 3 C5 2 2 2 2 1 9 B5—C B5 C1 C2 C3 C4 C5 ri 基点base point C1 1 0 0 0 0 1 rmax=9→C3
rmin=1→C1
dm(C3: C1)=7C2 2 1 0 2 2 7 C3 2 2 1 2 2 9 C4 2 0 0 1 2 5 C5 2 0 0 0 0 3 B6—C B6 C1 C2 C3 C4 C5 ri 基点base point C1 1 0 0 0 0 1 rmax=9→C4
rmin=1→C1
dm(C4: C1)=3C2 2 1 0 0 2 5 C3 2 2 1 0 2 7 C4 2 2 2 1 2 9 C5 2 0 0 0 1 3 B7—C B7 C1 C2 C3 C4 C5 ri 基点base point C1 1 0 0 0 0 1 rmax=7→C2、C4、C5
rmin=1→C1
dm(C2: C1)=3C2 2 1 2 1 1 7 C3 2 0 1 0 0 3 C4 2 1 2 1 1 7 C5 2 1 2 1 1 7 2.2.2 求出AHP间接判断矩阵
三标度比较矩阵并不能准确地反映各因素在某准则下的相对重要性程度，因此必须将其变换成具有层次分析法特点和性质的间接判断矩阵。首先，计算各因素的排序指数：
$$ r_i=\sum\limits_{j=1}^n b_{i j} \quad \mathrm{i}=1, 2, \cdots, n $$ 再找出最大排序指数rmax和最小排序指数rmin：
$$ \begin{aligned} & r_{\max }=\max _{1 \leq i \leq n}\left\{r_i\right\} \\ & r_{\min }=\min _{1 \leq i \leq n}\left\{r_i\right\} \end{aligned} $$ 以Bmax、Bmin分别表示与rmax、rmin对应的因素，则当选取Bmax、Bmin作为基点比较因素，并按9标度数值给出这个基点的相对重要程度dm后，利用下面的变换式可求得反映各因素间相对重要性程度的AHP间接判断矩阵：
$$ D=\left[\begin{array}{cccc} d_{11} & d_{12} & \cdots & d_{1 n} \\ d_{21} & d_{22} & \cdots & d_{2 n} \\ \vdots & \vdots & \vdots & \vdots \\ d_{n 1} & d_{n 2} & \cdots & d_m \end{array}\right]=\left(d_{i j}\right)_{n \times n} $$ $$ d_{i j}= \begin{cases}\frac{r_i-r_j}{r_{\max }-r_{\min }}\left(d_m-1\right)+1 & r_i-r_j \geq 0 \\ {\left[\frac{r_j-r_i}{r_{\max }-r_{\min }}\left(d_m-1\right)+1\right]^{-1}} & r_i-r_j<0\end{cases} $$ 采用ri计算式算得ri各值，列在每一比较矩阵的倒数第二列，rmax、rmin以及它们对应的Bmax、Bmin的基点相对重要性程度dm，列在每一比较矩阵的最后一列。然后利用dij计算式算得各个AHP间接判断矩阵如下：
A1—B A1 B1 B2 B3 B4 B5 B6 B7 权向量
weight vector一致性检验
consistency checkB1 1 4 1/4 1/3 3 2 1/2 0.1040 λmax=7.1952
C.I.=0.0325
R.I.=1.32
C.R.=0.0246B2 1/4 1 1/7 1/6 1/2 1/3 1/5 0.0308 B3 4 7 1 2 6 5 3 0.3517 B4 3 6 1/2 1 5 4 2 0.2412 B5 1/3 2 1/6 1/5 1 1/2 1/4 0.0449 B6 1/2 3 1/5 1/4 2 1 1/3 0.0678 B7 2 5 1/3 1/2 4 3 1 0.1596 B1—C B1 C1 C2 C3 C4 C5 权向量
weight vector一致性检验
consistency checkC1 1 2/5 7/2 5/2 1 0.2078 λmax=5.0602
C.I.=0.0150
R.I.=1.12
C.R.=0.0134C2 5/2 1 5 4 5/2 0.4250 C3 2/7 1/5 1 1/2 2/7 0.0618 C4 2/5 1/4 2 1 2/5 0.0976 C5 1 2/5 2/7 5/2 1 0.2078 B2—C B2 C1 C2 C3 C4 C5 权向量
weight vector一致性检验
consistency checkC1 1 3/2 2 2/3 1/2 0.1821 λmax=5.0596
C.I.=0.0149
R.I.=1.12
C.R.=0.0133C2 2/3 1 2/3 1/2 2/5 0.1122 C3 1/2 3/2 1 2/5 1/3 0.1149 C4 3/2 2 5/2 1 2/3 0.2513 C5 2 5/2 3 3/2 1 0.3395 B3—C B3 C1 C2 C3 C4 C5 权向量
weight vector一致性检验
consistency checkC1 1 4 3 5 2 0.4174 λmax=5.0682
C.I.=0.0170
R.I.=1.12
C.R.=0.0152C2 1/4 1 1/2 2 1/3 0.0975 C3 1/3 2 1 3 1/2 0.1602 C4 1/5 1/2 1/3 1 1/4 0.0615 C5 1/2 3 2 4 1 0.2634 B4—C B4 C1 C2 C3 C4 C5 权向量
weight vector一致性检验
consistency checkC1 1 2/5 1/2 2/3 1/3 0.0975 λmax=5.0143
C.I.=0.0036
R.I.=1.12
C.R.=0.0032C2 5/2 1 3/2 2 2/3 0.2506 C3 2 2/3 1 3/2 1/2 0.1816 C4 3/2 1/2 2/3 1 2/5 0.1317 C5 3 3/2 2 5/2 1 0.3386 B5—C B5 C1 C2 C3 C4 C5 权向量
weight vector一致性检验
consistency checkC1 1 2/11 1/7 1/4 2/5 0.0435 λmax=5.1464
C.I.=0.0366
R.I.=1.12
C.R.=0.0327C2 11/2 1 2/5 5/2 4 0.2656 C3 7 5/2 1 4 11/2 0.4707 C4 4 2/5 1/4 1 5/2 0.1431 C5 5/2 1/4 2/11 2/5 1 0.0771 B6—C B6 C1 C2 C3 C4 C5 权向量
weight vector一致性检验
consistency checkC1 1 1/2 2/5 1/3 2/3 0.0974 λmax=5.0143
C.I.=0.0036
R.I.=1.12
C.R.=0.0032C2 2 1 2/3 1/2 3/2 0.1817 C3 5/2 3/2 1 2/3 2 0.2506 C4 3 2 3/2 1 5/2 0.3386 C5 3/2 2/3 1/2 2/5 1 0.1317 B7—C B7 C1 C2 C3 C4 C5 权向量
weight vector一致性检验
consistency checkC1 1 1/3 3/5 1/3 1/3 0.0840 λmax=5.0080
C.I.=0.0020
R.I.=1.12
C.R.=0.0018C2 3 1 7/3 1 1 0.2654 C3 5/3 2/7 1 3/7 3/7 0.1198 C4 3 1 7/3 1 1 0.2654 C5 3 1 7/3 1 1 0.2654 2.3 由AHP间接判断矩阵计算权重[4, 8]
求解出间接判断矩阵的最大特征值λmax、C.I.及对应的特征量，将其归一化后即为某一层的有关因素相对于上一层相关因素的权重值。判断矩阵的一致性可用λmax、C.I.、R.I.和C.R.指标来检验，C.I.=(λmax-n)/(n-1)，R.I.按表 1确定，随机一致性比值C.R.=C.I./R.I.，当C. I.＜0.1时，则认为判断矩阵符合一致性要求。
表 1 平均一致性指标Table 1. The average consistency indicatorn 1 2 3 4 5 6 7 8 9 R.I. 0 0 0.58 0.90 1.12 1.24 1.32 1.41 1.45 用方根法求出各个间接判断矩阵的权向量和一致性检验指标，分别列在每个间接判断矩阵的后两列。然后求出5个监测点C1、C2、C3、C4和C5对于宜渔指标A1而言，相对优劣排序及一致性检验，见表 2。
表 2 A1—B—C层次总排序Table 2. The general arrangement for layers A1—B—CA1 B1
0.1040B2
0.0308B3
0.3517B4
0.2412B5
0.0449B6
0.0678B7
0.1596层次总排序
layers sequencing一致性检验
consistency checkC1 0.2078 0.1821 0.4174 0.0975 0.0435 0.0974 0.0840 0.2195 C.I.=0.0111
R.I.=1.12
C.R.=0.0099C2 0.4250 0.1122 0.0975 0.2506 0.2656 0.1817 0.2654 0.2091 C3 0.0618 0.1149 0.1602 0.1816 0.4707 0.2506 0.1198 0.1674 C4 0.0976 0.2513 0.0615 0.1317 0.1431 0.3386 0.2654 0.1430 C5 0.2078 0.3395 0.2634 0.3386 0.0771 0.1317 0.2654 0.2610 由表 2可见，相对于宜渔指标A1这个目标而言，5个监测点C1、C2、C3、C4和C5相对优劣排序为：C5＞C1＞C2＞C3＞C4。因此，E点宜渔指标最好。
按照上面方法，分析巢湖水质指标，计算出A2—B—C层次总排序，见表 3。
表 3 A2—B—C层次总排序Table 3. The general arrangement for layers A2—B—CA2 B1
0.5690B5
0.0523B6
0.2741B8
0.0523B9
0.0523层次总排序
layers sequncing一致性检验
consistency checkC1 0.1253 0.0435 0.0615 0.0615 0.0615 0.0969 C.I.=0.0169 R.I.=1.12 C.R.=0.0151 C2 0.0613 0.1431 0.1602 0.0975 0.2634 0.1051 C3 0.4213 0.0771 0.2634 0.1602 0.4174 0.3462 C4 0.2668 0.2656 0.4174 0.4174 0.1602 0.3103 C5 0.1253 0.4707 0.0975 0.2634 0.0975 0.1415 由表 3可见，相对于水质指标A2这个目标而言，5个监测点C1、C2、C3、C4和C5相对优劣排序为：C3＞C4＞C5＞C2＞C1。因此，C点水质指标最好。
最后，求出宜渔指标A1和水质指标A2对于巢湖渔业环境优劣总目标层M的层次的排序及一致性检验，见表 4。
表 4 M—A—B—C层次总排序Table 4. The general arrangement for layers A3—B—CM A1
0.35A2
0.65层次总排序
layers sequencing一致性检验
consistency checkC1 0.2195 0.0969 0.1398 C.I.=0.01149
R.I.=1.12
C.R.=0.0133C2 0.2091 0.1051 0.1416 C3 0.1674 0.3462 0.2836 C4 0.1430 0.3103 0.2517 C5 0.2610 0.1415 0.1833 由表 4可见，相对于巢湖渔业环境优劣总目标层M而言，通过综合宜渔指标A1和水质指标A2等多因素的评判，最后得出5个监测点相对优劣总排序为：C3＞C4＞C5＞C2＞C1。因此，巢湖渔业环境C3(即监测点C)点指标最好。
3. 讨论
巢湖渔业环境5个监测点，C点渔业环境相对最好。C点水体单元位于巢湖东湖湖心，外源性污染影响较小，水域环境相对稳定，该水域同其它水域之间存在着明显的差异。从C点的生物环境来看，该点的蓝藻数量亦最低；蓝藻数量低，其对其它浮游生物的抑制作用就小，该点的生物多样性就会增加，区域内的小生境就会较其它水域好。从叶绿素a监测的结果可以看出C点的含量最低(0.094 μg · L-1)，低于一般湖泊富营养化标准值(0.1 μg · L-1)。从C点的水质环境来看，监测的17个因子中，有14个优于渔业水质标准；有3个超标，分别是总氮、总磷和石油类，该域水质总体尚可。
巢湖A点渔业环境相对最差。从A点的生物环境来看，该点的浮游生物数量和生物量都最高，其蓝藻数量也是全湖中最高的。从A点的水质环境来看，A点在巢湖的西湖区，有着众多的入湖河口，如南淝河、派河、丰乐河、杭埠河等，其中南淝河、派河向巢湖排入大量的城市污水，也是导致巢湖富营养化的主要原因之一，在水质监测结果中，A点的水质超标因子数是最多的。
监测点D、E同处巢湖的东部，巢湖地处亚热带江淮平原，夏季主导风向为东南风，处上风口；D点采样点在东口门，距柘皋河口与裕溪河口较近，E点采样点距烔炀河口较近。B点处于巢湖南部，距马尾河口不远，同D点、E点水体单元在地理、气象、水文、生物环境等方面具有较显著的相似性，仅区别于入湖径流不同。层次分析的结果说明，D点渔业环境优于E点，E点优于B点。
因此，从AHP的分析结果可以看出，巢湖水域的渔业生态环境，东湖湖心最好，东湖近岸水域次之，西湖水域最差。
-
![]()
图 2 频率对狭鳕鱼糜介电常数的影响
Figure 2. Effect of frequency on dielectric constant of pollack surimi
![]()
图 3 温度对狭鳕鱼糜介电常数的影响
Figure 3. Effect of temperature on dielectric constant of pollack surimi
![]()
图 6 加热腔体内部电场强度分布
Figure 6. Distribution of electric field intensity in heating chamber
![]()
图 7 无线电波加热后鱼糜温度分布的比较
Figure 7. Comparison of temperature distribution in surimi tempered by radio frequency
![]()
图 8 无线电波加热过程中温度模拟值与实测值的比较
Figure 8. Comparison of simulated and measured temperature during radio frequency tempering
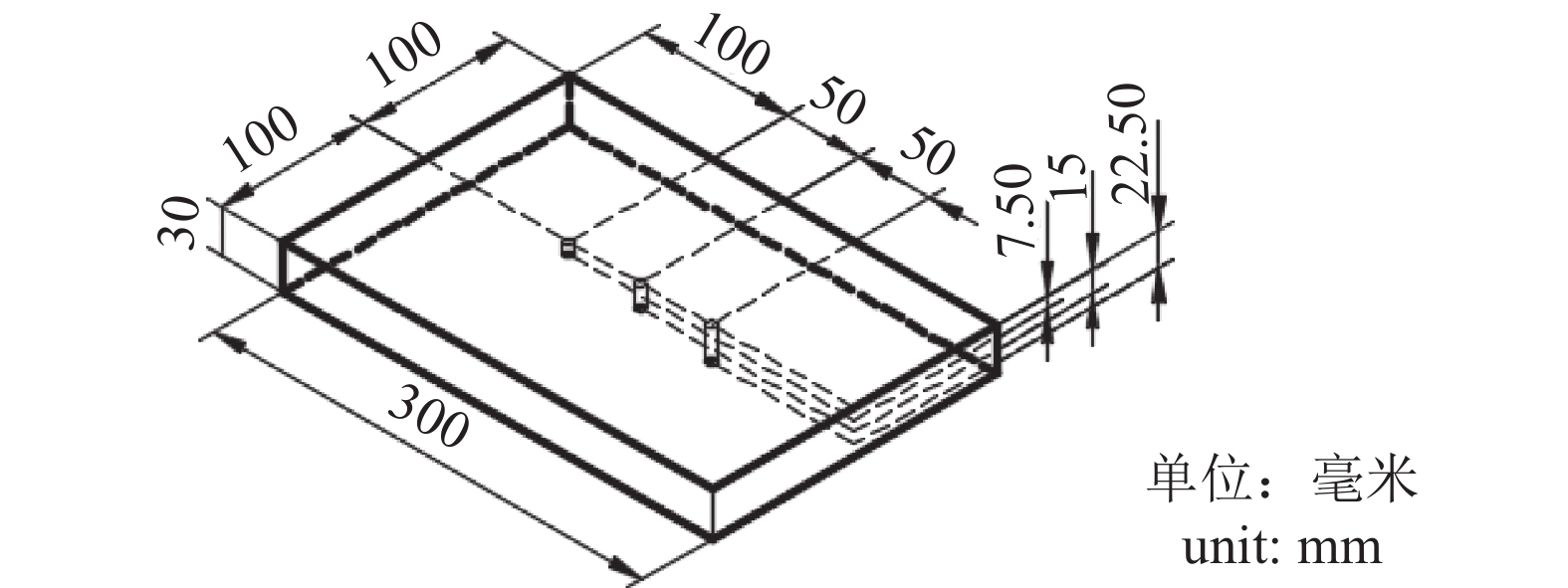
表 1 狭鳕鱼糜的热物性参数
Table 1 Thermophysical data of pollack surimi
参数
parameter数值或表达式
numerical or expression热导率/W·(m·K)–1
thermal conductivity0.536 密度/kg·m–3
density1 013 相对介电常数
relative dielectric constant0.000 8T 4 – 0.006 1T 3 – 0.38T 2 + 3.427 2T + 6.808 相对介电损失
relative dielectric loss– 0.002 1T 3 + 0.026 2T 2 + 2.397 6T + 26.705 比热容/J·(kg·K)–1
specific heat capacity4.085 初始温度/K
initial temperature255 上极板电势/V
upper plate potential8 000 下极板电势/V
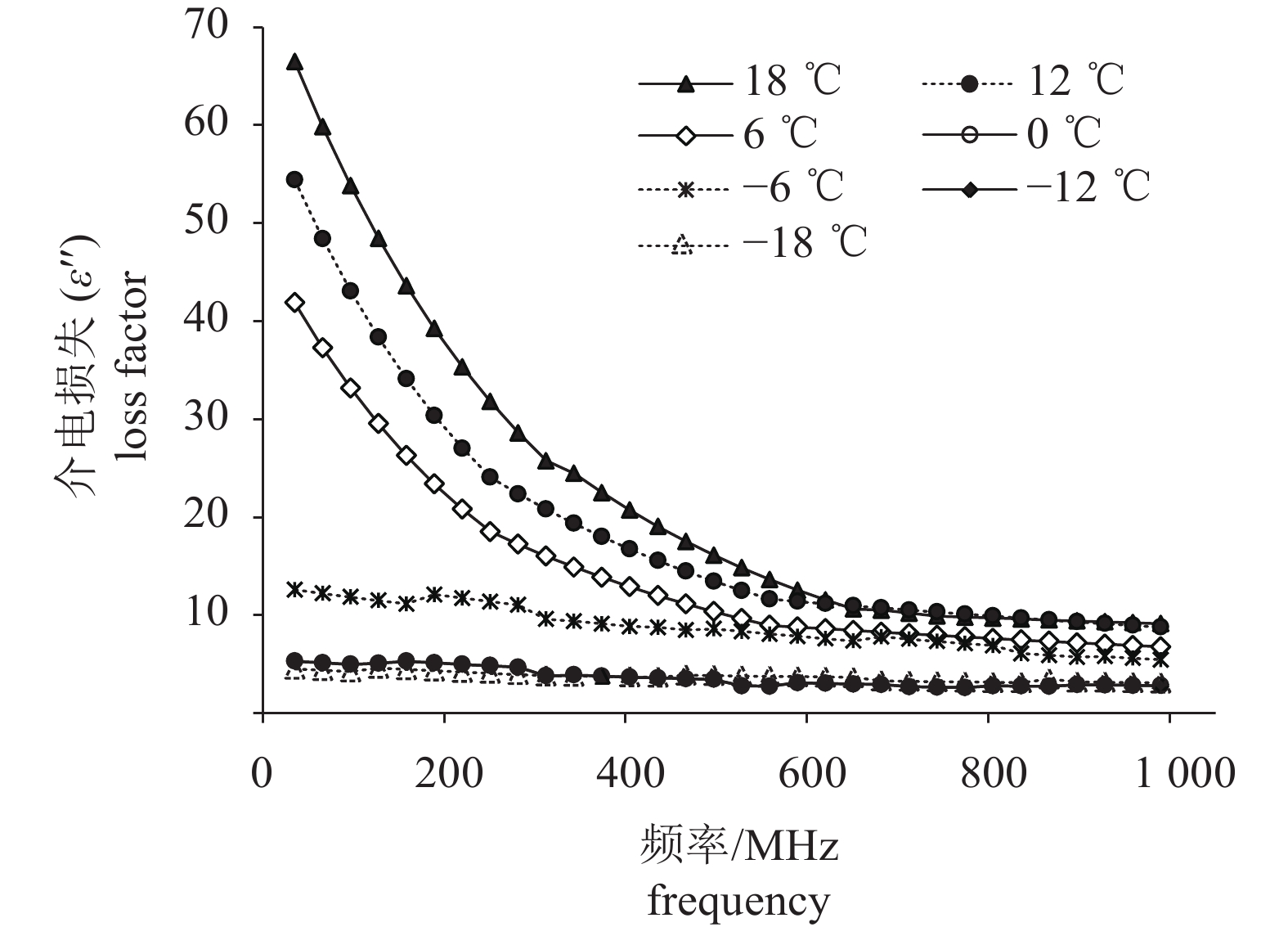
lower plate potential0 
下载: 导出CSV
表 2 27.1 MHz下狭鳕鱼糜介电特性拟合方程
Table 2 Predictive equations for dielectric properties of pollack surimi as a function (T) at 27.1 MHz
介电特性
dielectric property预测方程
predictive equationR2 $ {\varepsilon _r}'$ y = 0.000 8T 4 − 0.006 1T 3 − 0.38T 2 + 3.427 2T + 86.808 0.960 $ {\varepsilon _r}''$ y = – 0.002 1T 3 + 0.026 2T 2 + 2.397 6T + 26.705 0.998
下载: 导出CSV
-
[1] 曹燕, 程裕东. 鲢、狭鳕鱼糜微波加热凝胶形成的动力学分析[J]. 水产学报, 2005, 29(4): 547-551. [2] 阙婷婷, 刘文娟, 陈士国, 等. 水产品低温保鲜技术研究现状[J]. 中国食品学报, 2013, 13(8): 181-189. [3] BENLLOCH-TINOCO M, KAULMANN A, CORTE-REAL J, et al. Chlorophylls and carotenoids of kiwifruit puree are affected similarly or less by microwave than by conventional heat processing and storage[J]. Food Chem, 2015, 187: 254-262.
[4] CATHCART W H, PARKER J J. Defrosting frozen foods by high-frequency heat[J]. Food Res, 1946, 11(4): 341-344.
[5] ALFAIFI B, TANG J M, JIAO Y, et al. Radio frequency disinfestation treatments for dried fruit: model development and validation[J]. J Food Eng, 2014, 120: 268-276.
[6] PIYASENA P, DUSSAULT C, KOUTCHMA T, et al. Radio frequency heating of foods: principles, applications and related properties: a review[J]. Crit Rev Food Sci Nutr, 2003, 43(6): 587-606.
[7] LUAN D, WANG Y, TANG J, et al. Frequency distribution in domestic microwave ovens and its influence on heating pattern[J]. J Food Sci, 2017, 82(2): 429-436.
[8] 王冰冰. 虾肉糜的微波加热特性研究[D]. 上海: 上海海洋大学, 2016: 15-38. [9] 段续, 闫莎莎, 曾凡莲, 等. 基于介电特性的白蘑菇微波冻干传热传质模拟[J]. 现代食品科技, 2016, 32(6): 177-182. [10] 郭洁玉. 冷冻猪肉的射频解冻工艺[D]. 无锡: 江南大学, 2015: 25-40. [11] FARAG K W, LYNG J G, MORGAN D J, et al. A comparison of conventional and radio frequency thawing of beef meats: effects on product temperature distribution[J]. Food Bioproc Technol, 2011, 4(7): 1128-1136.
[12] UYAR R, ERDOGDU F, MARRA F. Effect of load volume on power absorption and temperature evolution during radio-frequency heating of meat cubes: a computational study[J]. Food Bioprod Proc, 2014, 92(C3): 243-251.
[13] CHEN L, HUANG Z, WANG K, et al. Simulation and validation of radio frequency heating with conveyor movement[J]. J Electromagnet Wav Appl, 2016, 30(4): 473-491.
[14] JIAO Y, TANG J, WANG S. A new strategy to imporve heating uniformity of low moisture foods in radio frequency treatment for pathogen control[J]. J Food Eng, 2014, 141: 128-138.
[15] LLAVE Y, TAKEMORI K, FUKUOKA M, et al. Mathematical modeling of shrinkage deformation in eggplant undergoing simultaneous heat and mass transfer during convection-oven roasting[J]. J Food Eng, 2016, 178: 124-136.
[16] ROMANO V, MARRA F. A numerical analysis of radio frequency heating of regular shaped foodstuff[J]. J Food Eng, 2008, 84(3): 449-457.
[17] UYAR R, BEDANE T F, ERDOGDU F, et al. Radio-frequency thawing of food products: a computational study[J]. J Food Eng, 2015, 146: 163-171.
[18] HUANG Z, MARRA F, WANG S J. A novel strategy for improving radio frequency heating uniformity of dry food products using computational modeling[J]. Innov Food Sci Emerging Technol, 2016, 34: 100-111.
[19] MARRA F, LYNG J, ROMANO V, et al. Radio-frequency heating of foodstuff: solution and validation of a mathematical model[J]. J Food Eng, 2007, 79(3): 998-1006.
[20] 贾敏, 薛长湖, 丛海花, 等. 频率和温度对鲍鱼介电特性的影响[J]. 食品工业科技, 2012, 33(18): 182-185, 198. [21] ALFAIFI M. Disinfestation of dried fruits using radio frequency energy[D]. Washington: Washington State University, 2013: 25-35.
[22] MARTIN-SÁNCHEZ A M, NAVARRO C, PÉREZ-ÁLVAREZ J A, et al. Alternatives for efficient and sustainable production of surimi: a review[J]. Comp Rev Food Sci Food Saf, 2009, 8(4): 359-374.
[23] 何天宝, 程裕东. 温度和频率对鱼糜介电特性的影响[J]. 水产学报, 2005, 29(2): 252-257. [24] TIWARI G, WANG S, TANG J, et al. Analysis of radio frequency (RF) power distribution in dry food materials[J]. J Food Eng, 2011, 104(4): 548-556.
[25] OZTURK S, KONG F, SINGH R K, et al. Radio frequency heating of corn flour: heating rate and uniformity[J]. Innov Food Sci Emer Technol, 2017, 44: 191-201.
[26] LIU S X, YU X Y, FUKUOKA M, et al. Modeling of fish boiling under microwave irradiation[J]. J Food Eng, 2014, 140: 9-18.
[27] CHEN F Y, WARNING A D, DATTA A K, et al. Thawing in a microwave cavity: comprehensive understanding of inverter and cycled heating[J]. J Food Eng, 2016, 180: 87-100.
[28] LUAN D, TANG J, PEDROW P D, et al. Analysis of electric field distribution within a microwave assisted thermal sterilization (MATS) system by computer simulation[J]. J Food Eng, 2016, 188: 87-97.
[29] CHEN J J, PITCHAI K, BIRLA S, et al. Modeling heat and mass transport during microwave heating of frozen food rotating on a turntable[J]. Food Bioprod Proc, 2016, 99: 116-127.
[30] PIAAZ A, PEDRIELLI R, BUSETTO M, et al. Use of radiofrequencies in the meat processing industry. Effects on the quality characteristics of meat and cooked meat products[J]. Ind Conserv, 1997, 72: 122-133.
-
期刊类型引用(2)
1. 李杰，张鹏，王腾，晏磊，杨炳忠，陈作志. 基于广义加性模型研究影响罩网沉降性能的因子. 南方水产科学. 2021(04): 74-81 . 
本站查看
2. 晏磊，李杰，杨炳忠，张鹏. 光诱罩网沉降性能对鸢乌贼渔获率的影响. 南方水产科学. 2018(04): 10-16 . 本站查看
其他类型引用(1)
计量
- 文章访问数:
- HTML全文浏览量:
- PDF下载量:
- 被引次数: 3
粤公网安备 44010502001741号
