Demographic analysis of scalloped hammerhead, Sphyrna lewini
-
摘要:
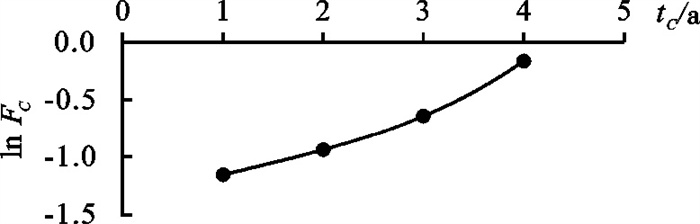
根据路氏双髻鲨Sphyrnalewini的平均性成熟年龄tα=5a、寿命tλ=15a、平均出生率b=6.4·a-1和自然死亡系数M=0.279·a-1, 估算在未开发状态下的内在瞬时增长率r0=0.265·a-1、世代生殖率R0=6.212、世代间期G=7·571 a和种群倍增时间tx2=2.616a;探讨了M对r0等种群统计参数的影响; 估算了不同开捕年龄tC时的临界捕捞死亡系数FC, 拟合了FC和tC的关系式, 证明FC随开捕年龄的增加而增加。当tC > 5a, 种群不会因捕捞而减少。
Abstract:Based on the mean age at first maturity tα=5 a, longevity tλ=15 a, average natality b=6.4·a -1, and natural mortality coefficient M=0.279·a -1, demographic analysis was used for estimating a population intrinsic increase coefficient (r0) of 0.265·a -1, a reproductive rate per generation (R0) of 6.212, a generation time (G) of 7.571 years and a population doubling time of 2.616 years under unfished. Demographic parameters such as r0 affected by M were discussed. FC at different first capture age were calculated, and relationship between FC and tC was fitted. The results indicated that FC increased with the first capture age. When tC > 5 a, the population will not decrease with capture.
-
Keywords:
- demographic analysis /
- Sphyrna lewini /
- scalloped hammerhead
-
鳗败血假单胞菌 (Pseudomonas anguilliseptica, PA) 于1972年由 Wakabayashi和Egusa[1]在日本鳗鲡 (Anguilla japonica) 上首次分离并报道,其后发现该菌主要影响欧洲地区的欧洲鳗鲡 (A. anguilla)[2]、大西洋鲷 (Sparus aurata)[3]、大西洋鳕 (Gadus morhua)[4]、圆鳍鱼 (Cyclopterus lumpus)[5]等海洋鱼类。因其感染的流行季节主要在水温低于12 ℃的冬季,且被感染宿主呈现体表出血性红斑的特征,因此被称为“冬季病”[6]和“红斑病”[7]。近年来,笔者研究发现PA是大口黑鲈 (Micropterus salmoides) 的一种新病原菌 (另文报道),对大口黑鲈的健康养殖造成威胁,但目前其在我国大口黑鲈中的流行情况尚不完全清楚。
PA的生长条件苛刻、培养时间长[8],同时其感染的临床症状与嗜水气单胞菌 (Aeromonas hydrophila)[9]、维氏气单胞菌 (A. veronii)[10]等相似,通过病原菌分离鉴定及临床症状的传统方法难以进行快速诊断,往往出现漏检、错检、误诊的情况,影响了PA感染的有效防控。建立快速、特异的检测方法不仅对PA的早期临床诊断和防控具有重要意义,还能为开展流行病学调查提供条件。目前针对水生动物细菌性病原的检测方法主要有环介导等温扩增技术 (LAMP)[11]、核酸序列依赖性扩增检测技术 (NASBA)[12]、免疫磁珠分离技术 (IMS)[13]、酶联免疫吸附测定技术 (ELISA)[14]、重组酶介导等温扩增技术 (RAA)[15]、实时荧光定量PCR (qPCR)[16]等,综合考虑经济性、操作简便性、时效性等因素后,本研究采用SYBR Green I 实时荧光定量PCR (SYBR Green I real-time quantitative PCR) 和重组酶介导等温扩增技术结合侧流层析试纸条(Recombinase-mediated isothermal amplification combined with lateral flow dipstick, RAA-LFD),以PA的管家基因recA为靶基因建立2种检测方法,以期为PA的早期、快速检测提供方法参考。
1. 材料与方法
1.1 主要材料与试剂
健康大口黑鲈 [外部无异常、PA特异性检测结果为阴性,体质量为 (18.4±1.5) g)]购自四川某苗种生产企业;各菌株 (表1) 由本实验室保存。主要试剂为:pUC18-T载体 (宝生物工程有限公司,大连);细菌基因组DNA提取试剂盒、胶回收试剂盒及质粒提取试剂盒 (天根生化科技有限公司,北京);Taq Pro Universal SYBR qPCR Master Mix、2×Rapid Taq Master Mix (诺唯赞生物科技有限公司,南京);RAA核酸扩增试剂盒及侧流层析试纸条 (乐尚生物科技有限公司,无锡)。
表 1 研究所用的细菌菌株Table 1 Bacterial strains used in this study菌株名称
Bacterial strain菌株编号
Strain No.鳗败血假单胞菌 Pseudomonas anguilliseptica SICAU PA001 无乳链球菌 Streptococcus agalactiae GY104 拟态弧菌 Vibrio mimicus SCCF01 嗜水气单胞菌 Aeromonas hydrophila YYL 鮰爱德华菌 Edwardsiella ictaluri CNHYC01 鰤诺卡菌 Nocardia seriolae DY01 荧光假单胞菌 P. fluorescens ATCC® 13525 柱状黄杆菌 Flavobacterium cloumnare ATCC® 23463 维氏气单胞菌 A. veronii ATCC® 35624 1.2 方法
1.2.1 引物及探针的设计、合成与特异性检测
根据Mjølnerød等[17]对53株PA管家基因的分析结果,选择其中具有完全同源性的recA基因 (登录号:MW685241.1—MW685293.1) 作为建立2种检测方法的靶基因,并采用验证recA基因的特异性引物 (recA-PCR-F/R) 作为本研究PCR和构建标准品质粒的引物。使用Primer Premier 5.0软件,根据SYBR Green I real-time quantitative PCR和RAA引物设计原则设计多对引物,并设计RAA探针。成功筛选出引物recA-qPCR-F/R、recA-RAA-F/R和探针recA-RAA-Probe,并在recA-RAA-R的5' 端标记生物素。本研究所用引物和探针 (表2) 均由生工生物工程 (上海) 有限公司合成。将recA-RAA-F/R配合recA-RAA-Probe的RAA扩增产物和recA-qPCR-F/R的PCR 扩增产物送至生工生物工程 (上海) 有限公司测序,在GenBank中获取相应序列并与测序结果进行比对,以验证引物的特异性。
表 2 研究所用引物及探针Table 2 Primers used in this study检测方法
Detection method引物名称
Primer name序列 (5'—3')
Sequence (5'−3')产物大小
Length of product/bpPCR recA-PCR-F/R F: CTCGCTTGGTCTGGACATCG
R: CCTTGCCTTGGCCGATCTT740 qPCR recA-qPCR-F/R F: GGCAGCTCTTACACCCAAAG
R: GGCTCCCAAACATAACACCTAT179 RAA-LFD recA-RAA-F/R F: CATGCACTTGACCCAATCTACGCAGCAAAG
R: Biotin CCCGTAATCTTACGCATTGCCTGACTCATC251 recA-RAA-Probe FAM AAGCTGAAATCGAAGGTGAAATTGGGGATA/idSp/
GCACGTTGGGTTGGC C3 Spacer1.2.2 标准品质粒的制备
以PA的基因组DNA为模板,用recA-PCR-F/R引物扩增recA基因目的片段,与pUC18-T载体连接后转化于DH5α感受态细胞,获得重组标准品质粒pUC18-recA。用微量核酸蛋白检测仪测定质粒浓度和纯度,并根据摩尔定律计算出单位体积质粒所含的DNA拷贝数。
1.2.3 qPCR和RAA扩增条件的优化
2种方法均采用2.816×106 拷贝·μL−1的标准品质粒进行优化。qPCR引物终浓度梯度设置为0.2~1.0 μmol·L−1,退火温度梯度为 58~64 ℃,以获得qPCR最小的平均Ct值和标准差;RAA引物终浓度梯度设置为 6.25~200 μmol·L−1,RAA扩增反应温度梯度为29~54 ℃,并通过观察不同条件下侧流层析试纸条第30分钟时的显色情况确定RAA扩增的最佳反应体系和反应条件。
1.2.4 qPCR和RAA-LFD检测方法的建立
qPCR方法的建立:将标准品质粒10倍稀释成9组浓度梯度 (2.816×109~2.816×101 拷贝·μL−1),采用优化后的反应条件 (反应体系总体积10 μL:recA-qPCR-F/R各0.2 μL,2×Taq Pro Universal SYBR qPCR Mix 5 μL,ddH2O 3.6 μL,模板DNA 1 μL;反应条件为:95 ℃预变性30 s;95 ℃变性10 s,60 ℃退火20 s,72 ℃延伸10 s,40个循环) 进行qPCR检测,建立以质粒拷贝数对数值为x轴和以Ct值为y轴的qPCR标准曲线;每个浓度梯度的标准品质粒设置3次qPCR检测,以进行组内重复性分析,并以相同的标准品质粒为模板,在不同时间点开展3次独立重复实验,进行组间重复性分析;通过Ct值变异系数评价方法的重复性。
RAA-LFD方法的建立:采用优化后的反应条件 (反应体系总体积50 μL:recA-RAA-Probe 0.6 μL,recA-RAA-LF/LR各2.1 μL,基础缓冲液25 μL,模板DNA 2 μL,ddH2O 15.2 μL;各组分混匀后,加入含冻干酶粉的反应管中溶解,然后加入280 mmol·L−1的醋酸镁溶液3 μL作为启动剂混匀,39 ℃水浴20 min,立即置于冰上5 min) 对标准品质粒 (2.816×109 拷贝·μL−1) 进行RAA扩增,将侧流层析试纸条样品垫末端垂直插入含有50 μL稀释5倍的RAA核酸扩增产物的EP管中,观察试纸条C线和T线在30 min内的颜色变化,并参考Saxena等[18]的标准判定结果。
1.2.5 qPCR和RAA-LFD灵敏性
各取101~108 拷贝·μL−1的标准品质粒1 μL作为模板,分别进行qPCR、RAA和普通PCR检测,分析3种方法的灵敏性。
1.2.6 qPCR和RAA-LFD特异性
取质量浓度为10 ng·μL−1的各株细菌DNA 1 μL作为模板,同时进行qPCR、RAA和普通PCR扩增,分析3种方法的特异性。
1.2.7 qPCR和RAA-LFD方法的应用
通过建立的qPCR和RAA-LFD方法以及普通PCR方法,对10份疑似感染PA的大口黑鲈肾组织样本与30份腹腔注射感染PA的大口黑鲈肾组织样本进行检测并比较检出率的差异。
选取15尾健康大口黑鲈 (共3组,每组5尾),腹腔注射PA,于感染后的第7天分别采集每组病鱼的肝、脾、肾、心、肌肉、脑、眼和鳃组织并提取DNA,采用qPCR方法和标准曲线方程测定每组病鱼组织中的平均PA载量。
2. 结果
2.1 引物及探针的特异性
PA提取的DNA通过qPCR引物recA-qPCR-F/R扩增后得到179 bp的单一目的条带 (图1-a),通过RAA引物recA-RAA-F/R配合recA-RAA-Probe扩增后得到251 bp的单一目的条带 (图1-b)。将目的条带的测序结果与GenBank中获取的相应序列进行比对,结果显示2段产物序列与其他序列的同源性均为100% (图2)。
![]() 图 1 基于鳗败血假单胞菌recA基因的引物特异性与标准品质粒鉴定结果注:a. qPCR引物特异性结果;b. RAA引物及探针特异性结果;c. 标准品质粒鉴定结果。Fig. 1 Results based on primers specificity and standard plasmid identification of recA gene of P. anguillisepticaNote: a. Primer specificity results for qPCR; b. Primer and probe specificity results for RAA; c. Results of standard plasmid identification.
图 1 基于鳗败血假单胞菌recA基因的引物特异性与标准品质粒鉴定结果注:a. qPCR引物特异性结果;b. RAA引物及探针特异性结果;c. 标准品质粒鉴定结果。Fig. 1 Results based on primers specificity and standard plasmid identification of recA gene of P. anguillisepticaNote: a. Primer specificity results for qPCR; b. Primer and probe specificity results for RAA; c. Results of standard plasmid identification.2.2 标准品质粒的制备
标准品质粒扩增获得的目的片段大小为740 bp (图1-c),与预期大小相符。重组质粒pUC18-recA的质量浓度为1.057×102 ng·μL−1,经质粒拷贝数计算公式换算后为2.816×1010 拷贝·μL−1。
2.3 qPCR和RAA扩增条件的优化
qPCR扩增条件的优化结果显示,qPCR引物浓度为0.6 μmol·L−1、退火温度为60 ℃时平均Ct值 (13.259) 和标准差 (0.045) 最小 (表3),故选择该引物浓度和退火温度为最佳反应条件。
表 3 鳗败血假单胞菌qPCR扩增条件的优化Table 3 Optimization of qPCR amplification conditions for P. anguilliseptica退火温度
Annealing temperature/℃引物浓度
Primer concentration/(μmol·L−1)Ct值
Ct value平均Ct值
Mean Ct value标准差
Standard deviation58 0.2 14.583 15.418 14.845 14.949 0.349 0.4 13.876 14.356 14.230 14.154 0.203 0.6 13.336 13.576 13.429 13.447 0.099 0.8 13.745 13.401 13.313 13.486 0.187 1.0 13.756 13.444 13.895 13.698 0.189 60 0.2 14.419 15.074 14.355 14.616 0.325 0.4 14.079 13.832 13.786 13.899 0.129 0.6 13.204 13.259 13.314 13.259 0.045 0.8 13.349 13.608 13.573 13.510 0.115 1.0 13.749 14.023 13.988 13.920 0.122 62 0.2 16.812 16.033 16.267 16.370 0.326 0.4 15.305 15.466 15.077 15.283 0.160 0.6 14.275 14.119 14.439 14.277 0.131 0.8 14.206 14.401 15.046 14.551 0.359 1.0 14.748 14.207 14.805 14.587 0.269 64 0.2 15.745 16.784 15.695 16.075 0.502 0.4 14.139 14.916 14.878 14.644 0.358 0.6 15.061 15.328 15.457 15.282 0.165 0.8 15.740 15.112 15.557 15.470 0.264 1.0 15.963 15.369 15.844 15.725 0.257 RAA扩增条件的优化结果如图3所示。试纸条显色第30分钟时的T线灰度值与引物浓度成正比 (图3-a—3-b),由于引物浓度过高可能会产生大量引物二聚体,且在25~100 μmol·L−1时T线灰度值差异不显著 (p>0.05),故选择50 μmol·L−1为最佳引物浓度。T线灰度值在反应温度介于29~54 ℃时呈正态分布,在39~44 ℃时达到最高,差异不显著 (p>0.05,图3-c—3-d),故选择其中获得最高T线灰度值的39 ℃为最适反应温度。
![]() 图 3 鳗败血假单胞菌RAA扩增条件的优化注:a. 不同引物浓度的RAA-LFD显色结果;b. 灰度分析结果 (对应3-a);c. 不同反应温度的RAA-LFD显色结果;d. 灰度分析结果 (对应3-c);不同字母表示差异显著 (p<0.05)。Fig. 3 Optimization of RAA amplification conditions for P. anguillisepticaNote: a. Results of RAA-LFD color development with different primer concentrations; b. Grayscale analysis results (Corresponding to 3-a); c. Results of RAA-LFD color development at different reaction temperatures; d. Grayscale analysis results (Corresponding to 3-c); different letters represent significant differences (p<0.05).
图 3 鳗败血假单胞菌RAA扩增条件的优化注:a. 不同引物浓度的RAA-LFD显色结果;b. 灰度分析结果 (对应3-a);c. 不同反应温度的RAA-LFD显色结果;d. 灰度分析结果 (对应3-c);不同字母表示差异显著 (p<0.05)。Fig. 3 Optimization of RAA amplification conditions for P. anguillisepticaNote: a. Results of RAA-LFD color development with different primer concentrations; b. Grayscale analysis results (Corresponding to 3-a); c. Results of RAA-LFD color development at different reaction temperatures; d. Grayscale analysis results (Corresponding to 3-c); different letters represent significant differences (p<0.05).2.4 qPCR和RAA-LFD检测方法的建立
采用优化后的反应条件对9组10倍梯度稀释的标准品质粒 (2.816×109~2.816×101 拷贝·μL−1) 进行qPCR检测,建立质粒拷贝数对数值与Ct值对应关系的定量标准曲线 (图4-a),标准曲线介于2.816×107~2.816×102 拷贝·μL−1之间Ct值线性良好。标准曲线方程为y= –3.158x+32.213,r2=0.999 2。熔解曲线显示,6个浓度的标准品质粒在熔解温度为 (84±0.5) ℃时均出现特异性细窄单峰 (图4-b),表明扩增产物具有良好的特异性和均一性。标准曲线重复性检测结果表明,组内变异系数≤0.730%,组间变异系数≤1.443% (表4),该方法重复性和稳定性较好。
采用优化后的反应条件对标准品质粒 (2.816×109 拷贝·μL−1) 进行RAA扩增,并通过侧流层析试纸条对扩增产物进行检测。结果显示,T线灰度值与显色时长成正比,在15 min后试纸条T线显色情况较好 (T线灰度值≥40.0),且各时间点无显著性差异 (p>0.05,图5-a—5-b),表明该方法最快15 min可读取检测结果,且显色较为稳定。
表 4 鳗败血假单胞菌qPCR方法重复性试验结果Table 4 Results of qPCR reproducibility test for P. anguilliseptica标准品质粒浓度
Standard quality plasmid
concentration/
(拷贝·μL−1)组内重复性试验
Repeatability test within group组间重复性试验
Repeatability test between group平均Ct值±标准差
Mean Ct value±standard deviation变异系数
Variable coefficient/%平均Ct值±标准差
Mean Ct value±standard deviation变异系数
Variable coefficient/%2.816×107 10.300±0.032 0.311 10.328±0.149 1.443 2.816×106 13.213±0.042 0.318 13.257±0.122 0.920 2.816×105 16.710±0.122 0.730 16.606±0.212 1.277 2.816×104 19.511±0.102 0.523 19.523±0.111 0.569 2.816×103 22.609±0.081 0.358 22.618±0.168 0.743 2.816×102 26.083±0.130 0.498 26.120±0.210 0.804 ![]() 图 5 鳗败血假单胞菌RAA-LFD反应结果注:a. 不同反应时间的RAA-LFD显色;b. 灰度分析 (对应5-a),不同字母表示差异显著 (p<0.05)。Fig. 5 Results of RAA-LFD reaction of P. anguillisepticaNote: a. Results of RAA-LFD color development at different reaction time; b. Grayscale analysis results (Corresponding to 5-a), different letters represent significant differences (p<0.05).
图 5 鳗败血假单胞菌RAA-LFD反应结果注:a. 不同反应时间的RAA-LFD显色;b. 灰度分析 (对应5-a),不同字母表示差异显著 (p<0.05)。Fig. 5 Results of RAA-LFD reaction of P. anguillisepticaNote: a. Results of RAA-LFD color development at different reaction time; b. Grayscale analysis results (Corresponding to 5-a), different letters represent significant differences (p<0.05).2.5 qPCR和RAA-LFD的灵敏性
由普通PCR灵敏性试验结果可知,模板浓度低于2.816×105 拷贝·μL−1时,无法产生扩增条带 (图6-a),故普通PCR的最低检测浓度为2.816×105 拷贝·μL−1。由2.816×107 拷贝·μL−1浓度起始的6个10倍梯度稀释的标准品质粒扩增曲线可知,模板浓度在低于2.816×102 拷贝·μL−1时,无法产生扩增曲线 (图6-b),故qPCR的最低检测浓度为2.816×102 拷贝·μL−1。由RAA-LFD灵敏性试验结果可知,模板浓度低于2.816×104 拷贝·μL−1时扩增产物在试纸条上显色极不明显 (T线灰度值≤5.0,图6-c—6-d),故RAA-LFD的最低检测浓度为2.816×104 拷贝·μL−1。综上,构建的2种方法灵敏性均高于普通PCR,且qPCR方法的灵敏性最高。
![]() 图 6 鳗败血假单胞菌灵敏性试验结果注:a. 普通PCR灵敏性电泳图,M. 标准分子量DL 2000,NC. 阴性对照,1. 2.816×108 拷贝·μL−1,2. 2.816×107 拷贝·μL−1,3. 2.816×106拷贝·μL−1,4. 2.816×105 拷贝·μL−1,5. 2.816×104 拷贝·μL−1;b. qPCR标准质粒扩增曲线,1—6. 质粒标准品浓度为2.816×107~2.816×102 拷贝·μL−1;c. 不同浓度标准质粒的RAA-LFD显色;d. 灰度分析 (对应6-c),不同字母表示差异显著 (p<0.05)。Fig. 6 Results of sensitivity test for P. anguillisepticaNote: a. Sensitive electrophoresis pattern of PCR, M. Standard molecular weight is DL 2000, NC. Negative control, 1. 2.816×108 copies·μL−1, 2. 2.816×107 copies·μL−1, 3. 2.816×106copies·μL−1, 4. 2.816×105 copies·μL−1, 5. 2.816×104 copies·μL−1; b. Amplification curves of qPCR standard plasmids, 1−6. Concentration of standard plasmid was 2.816×107−2.816×102 copies·μL−1; c. Results of RAA-LFD color development with different concentrations of standard plasmids; d. Grayscale analysis results (Corresponding to 6-c), different letters represent significant differences (p<0.05).
图 6 鳗败血假单胞菌灵敏性试验结果注:a. 普通PCR灵敏性电泳图,M. 标准分子量DL 2000,NC. 阴性对照,1. 2.816×108 拷贝·μL−1,2. 2.816×107 拷贝·μL−1,3. 2.816×106拷贝·μL−1,4. 2.816×105 拷贝·μL−1,5. 2.816×104 拷贝·μL−1;b. qPCR标准质粒扩增曲线,1—6. 质粒标准品浓度为2.816×107~2.816×102 拷贝·μL−1;c. 不同浓度标准质粒的RAA-LFD显色;d. 灰度分析 (对应6-c),不同字母表示差异显著 (p<0.05)。Fig. 6 Results of sensitivity test for P. anguillisepticaNote: a. Sensitive electrophoresis pattern of PCR, M. Standard molecular weight is DL 2000, NC. Negative control, 1. 2.816×108 copies·μL−1, 2. 2.816×107 copies·μL−1, 3. 2.816×106copies·μL−1, 4. 2.816×105 copies·μL−1, 5. 2.816×104 copies·μL−1; b. Amplification curves of qPCR standard plasmids, 1−6. Concentration of standard plasmid was 2.816×107−2.816×102 copies·μL−1; c. Results of RAA-LFD color development with different concentrations of standard plasmids; d. Grayscale analysis results (Corresponding to 6-c), different letters represent significant differences (p<0.05).2.6 qPCR和RAA-LFD的特异性
qPCR特异性扩增曲线结果显示,PA的DNA扩增结果为阳性,而其他8株菌的结果为阴性 (图7-a)。RAA-LFD特异性试验结果显示,PA的DNA扩增产物显色较为明显 (T线灰度值为27.0),而其他8株菌显色极不明显 (T线灰度值≤3.0,图7-b—7-c)。普通PCR特异性试验结果显示,PA的DNA扩增后均出现单一条带(740 bp),而其他8株菌DNA均未出现扩增条带 (图7-d)。综上,3种检测方法均出现明显的特异性扩增结果。
![]() 图 7 鳗败血假单胞菌特异性试验结果注:a. qPCR特异性扩增曲线;b. 不同菌株的RAA-LFD显色结果;c. 灰度分析结果 (对应7-b),不同字母表示差异显著 (p<0.05);d. 普通PCR特异性电泳图。Fig. 7 Results of specificity test for P. anguillisepticaNote: a. Specific amplification curves of qPCR; b. RAA-LFD chromogenic results of different strains; c. Grayscale analysis results (Corresponding to 7-b), different letters represent significant differences (p<0.05); d. Specific electrophoresis pattern of PCR.
图 7 鳗败血假单胞菌特异性试验结果注:a. qPCR特异性扩增曲线;b. 不同菌株的RAA-LFD显色结果;c. 灰度分析结果 (对应7-b),不同字母表示差异显著 (p<0.05);d. 普通PCR特异性电泳图。Fig. 7 Results of specificity test for P. anguillisepticaNote: a. Specific amplification curves of qPCR; b. RAA-LFD chromogenic results of different strains; c. Grayscale analysis results (Corresponding to 7-b), different letters represent significant differences (p<0.05); d. Specific electrophoresis pattern of PCR.2.7 qPCR和RAA-LFD方法的应用
通过建立的2种方法及普通PCR方法对采集的10份疑似感染PA的大口黑鲈肾组织样本与30份腹腔注射感染PA的大口黑鲈肾组织样本进行检测。普通PCR方法的阳性样本检出率为62.50%,而qPCR和RAA-LFD方法的阳性样本检出率分别为87.50%和85.00% (表5),较普通PCR方法有明显提高。且qPCR与RAA-LFD方法具有高一致性,较普通PCR方法更可靠。
表 5 鳗败血假单胞菌3种检测方法的应用结果对比Table 5 Comparison of application results of three detection methods for P. anguilliseptica检测方法
Detection method样本总数
Total sample number阳性样本检出率
Detection rate of positive samples共同阳性/阴性样本数
Number of common positive/negative samplesKappa值
Kappa valueqPCR/RAA-LFD 40/40 87.50%/85.00% 34/5 0.895 qPCR/PCR 40/40 87.50%/62.50% 25/5 0.385 RAA-LFD/PCR 40/40 85.00%/62.50% 25/6 0.455 注:Kappa值的取值范围为0~1.00,其中1.00表示完全一致,0表示完全不一致。常见标准为:Kappa值在0~0.20表示一致性极低,0.21~0.40表示一致性较低,0.41~0.60表示一致性中等,0.61~0.80表示一致性较高,0.81~1.00表示一致性极高。 Note: The Kappa values ranged from 0 to 1.00, with 1.00 indicating complete agreement and 0 indicating complete disagreement. Common criteria are as follows: Kappa of 0−0.20 represents very low consistency; 0.21−0.40 represents low consistency; 0.41−0.60 represents medium consistency; 0.61−0.80 represents high consistency; 0.81−1.00 represents extremely high consistency. 使用qPCR方法测得PA载量 (图8),PA在大口黑鲈肾中载量最高,达3.533×107 拷贝·ng−1,在PA总载量中占比达70.84%;在肝、脾、眼和脑等组织器官中的载量均较高,但在鳃中载量极低。
3. 讨论
PA曾被认为是海水鱼类的重要病原菌,近年来其感染谱有向淡水鱼类扩展的趋势。Ajengaekanurmaningdyah和Kurniasih[19]于2018年首次报道了PA感染淡水鱼类金鱼 (Cyprinus carpio) 引起死亡;本实验室近年发现PA已成为危害大口黑鲈的新细菌性病原,给大口黑鲈养殖产业造成严重威胁 (另文报道)。PA感染后病鱼处于无明显症状的亚临床期较长,发展到明显症状的临床期往往导致高死亡率,由于PA仅在TSA和BHI等营养丰富的平板上生长[8],且出现菌落的时间超过1周,采用传统病原菌分离鉴定及临床症状进行诊断的难度大、效率低,建立快速、特异、灵敏的PA检测方法在该病的防控中就显得尤为重要。目前,国内外对于PA检测方法的研究较少,除了传统的分离鉴定外,有采用普通PCR进行检测的报道[20-21],但在笔者实验室的应用中发现普通PCR存在假阴性率高的缺点。
qPCR方法具有重复性好、特异性强和灵敏性高等优点[22]。相较于TaqMan探针法,SYBR Green I法只需在反应混合液中加入引物和待测样品的DNA,无需设计合成探针,简单高效且成本较低[23],目前已应用于鼠伤寒沙门氏菌 (Salmonella typhimurium)[24]、金黄色葡萄球菌 (Staphylococcus aureus)[25]等病原菌的检测。RAA方法对比NASBA、LAMP等温扩增方法有扩增温度较低、仅需1对引物和探针、反应时间较短的优势[26-27],已在铜绿假单胞菌 (Pseudomonas aeruginosa)[28]、鰤诺卡菌 (Nocardia seriolae)[29]等病原菌的检测上得到了应用。RAA-LFD 方法则更利于脱离实验室环境,应用到现场检测中,目前该技术已在副结核分枝杆菌 (Mycobacterium avium subsp. paratuberculosis)[30]、霍乱弧菌 (Vibrio cholerae)[31]、停乳链球菌 (Streptococcus dysgalactiae)[32]等病原菌上开展了研究,为这些病原菌的现场快速检测提供了重要的支持。本研究基于PA管家基因recA成功建立了SYBR Green I real-time quantitative PCR和RAA-LFD 2种快速、特异的检测方法,检出率相较PCR方法提高了至少22.50%。qPCR方法的最低检测浓度为2.816×102 拷贝·μL−1,RAA-LFD方法的最低检测浓度为2.816×104 拷贝·μL−1,尽管建立的qPCR方法的灵敏性高于RAA-LFD方法,但2种方法检测结果的一致性较高,且RAA-LFD方法更具有开展现场检测的优势。这2种方法的建立为PA的早期快速检测与流行病学调查提供了重要的方法依据。
据报道,PA感染金鱼致肾、肝、脾、肌肉、心、脑等组织器官病变,且肾的病变最为严重,表现为严重坏死[19];PA感染比目鱼 (Psetta maxima) 可致肾、肝、脾、肌肉、脑、眼等组织器官出现病变[33]。由此可见PA感染宿主致全身多组织器官损伤,但对于这些组织器官出现的病变与病原菌的分布及载量之间的关系尚不清楚。本研究建立的SYBR Green I real-time quantitative PCR方法可对PA 进行定量检测,使用该方法对PA感染大口黑鲈后病原菌在各组织的分布与载量进行分析,结果发现PA感染后菌体在肾、肝、脾、眼、脑、心等全身多组织器官分布,其中在肾中的载量最高,其感染致宿主组织器官的病理损伤程度与病原菌的载量有直接关系,表明肾可能是PA感染最重要的侵袭靶器官。
-
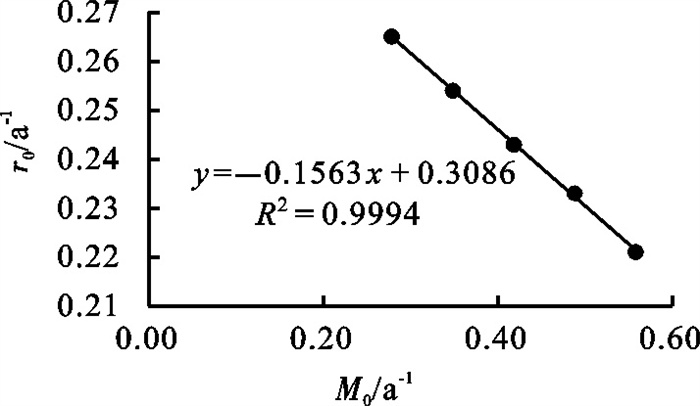
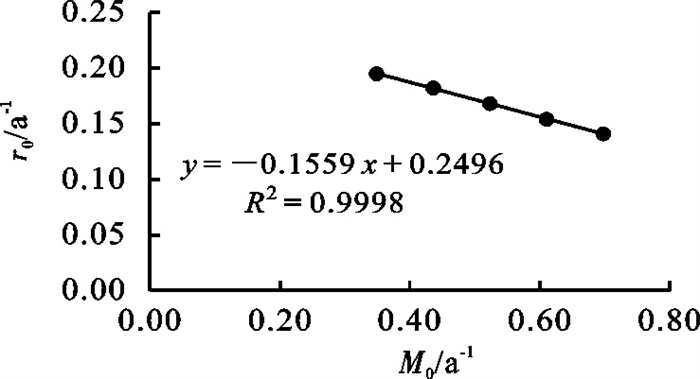
表 1 未开发状态下各种自然死亡对种群统计参数的影响
Table 1 Demographic parameters influenced by different natural mortality under unexploited
编号
no.tα/a tλ/a b/a-1 M0/a-1 M1~M15/a-1 r0/a-1 R0 G/a tx2/a I/% 1 5 15 6.4 0.279 0.279 0.265 6.212 7.571 2.616 30.3 2 5 15 6.4 0.349 0.279 0.254 5.792 7.571 2.729 28.9 3 5 15 6.4 0.419 0.279 0.243 5.401 7.571 2.852 27.5 4 5 15 6.4 0.488 0.279 0.233 5.041 7.571 2.975 26.2 5 5 15 6.4 0.558 0.279 0.221 4.700 7.571 3.136 24.7 6 5 15 6.4 0.349 0.349 0.195 3.712 7.152 3.555 21.5 7 5 15 6.4 0.436 0.349 0.182 3.403 7.152 3.809 20.0 8 5 15 6.4 0.524 0.349 0.168 3.116 7.152 4.126 18.3 9 5 15 6.4 0.611 0.349 0.154 2.857 7.152 4.501 16.6 10 5 15 6.4 0.698 0.349 0.141 2.619 7.152 4.916 15.1 -
[1] CAILLIETG M. Demography of the central California population of the leopard shark (Triakis semifasciate)[J]. Aust J Mar Freshw Res, 1992, 43(1): 183-193. doi: 10.1071/MF9920183
[2] AUD W, Smith S E. Ademographic method with population densi-ty compensation for estimating productivity and yield per recruit of the leopard shark (Triakis semifasciata)[J]. Can J Fish Aquat Sci, 1997, 54(2): 415-420. doi: 10.1139/f96-288
[3] SMINKEY TR, MUSICK J A. Demographic analysis of the sandbar shark, Carcharhinus plumbeus, in the western North Atlantic[J]. Fish Bull, 1996, 94(2): 341-347.
[4] BEERKIRCHER L, SHIVJI M, CORTÉS E. A Monte Carlo demo-graphic analysis of the silky shark (Carcharhinus falciformis): im-plications of gear selectivity[J]. Fish Bull, 2003, 101(1): 168-174.
[5] HOENIG J M, GRUBER S H. Life history patterns in the elasmo-branchs: implications for fisheries management[M] //Pratt HL, Jr., Gruber S H, Taniuchi T. Elasmobranchs as living resources: Advances in the biology, ecology systematics, and the status of the fisheries. [S.l. ]: US Dep Commer, NOAA Tech Rep NMFS, 1990, 90: 1-16.
[6] SIMPFENDORFER C A. Mortality estimates and demographic anal-ysis for the Australian sharpnose shark, Rhizoprionodon taylori, from northern Australia[J]. Fish Bull, 1999, 97(4): 978-986.
[7] CORTÉS E. Demographic analysis of the Atlantic sharpnose shark Rhiyoprionodon terraenovaein the Gulf of Mexico[J]. Fish Bull, 1995, 93(1): 57-66.
[8] LIUK M, CHEN C T. Demographic analysis of the scalloped ham-merhead Sphyrna lewini, in the Northwestern Pacific[J]. Fish Sci, 1999, 65(2): 218-223. doi: 10.2331/fishsci.65.218
[9] CORTÉS E, PARSONS R. Comparative demography of two popula-tions of the bonnethead shark (Sphyrna tiburo)[J]. Can J Fish Aquat Sci, 1996, 53(4): 709-718. doi: 10.1139/f95-240
[10] XIAO Y, WALKER T L. Demographic analysis of gummy shark (Mustelus antarcticus) and school shark (Galeorhinus galeus) off southern Australia by applying a generalized Lotka equation and its dual equation[J]. Can J Fish Aquat Sci, 2000, 57(1): 214-222. doi: 10.1139/f99-224
[11] CAILLIETG M, MOLLETHF, PITTENGER G P, et al. Growth and demography of the Pacific angel shark (Squatina California), based upon tag returns off California[J]. Aust J Mar Freshw Res, 1992, 43(5): 1313-1330. doi: 10.1071/MF9921313
[12] SMITHS E, AU D W, SNOW C. Intrinsic rebound potentials of26species of Pacific sharks[J]. Aust J Mar FreshwRes, 1998, 49(8): 663-678. doi: 10.1071/MF97135
[13] BRANSTETTER S. Age, growth and reproductive biology of the silky shark, Carcharhinus falciformis, and the scalloped hammer-head, Sphyrna lewini, from the northwestern Gulf of Mexico[J]. Environ Biol Fishes, 1987, 19(3): 161-173. doi: 10.1007/BF00005346
[14] CHEN C T, LEU T C, JOUNG S J, et al. Age and growth of the scalloped hammerhead, Sphyrna lewini, in northeastern Taiwan waters[J]. Pacific Sci, 1990, 44(1): 156-170.
[15] CHEN C T, LEU T C, JOUNG S J. Notes on reproduction in the scalloped hammerhead, Sphyrna lewini, in northeastern Taiwan waters[J]. Fish Bull, 1988, 86(2): 398-393.
[16] CLARKE T A. The ecology of the scalloped hammerhead shark, Sphyrna lewini[J]. Pacific Sci, 1971, 25(1): 133-144.
[17] HOENIG J M. Empirical use of longevity data to estimate mortality rates[J]. Fish Bull, 1983, 81(4): 898-903.
[18] SOUTHWOOD TR E. Ecological methods with particular reference to the study of insect population[M]. [S.l. ]: The English Language Book Society and Chapman and Hall, 1978: 524. doi: 10.1007/978-94-009-1225-0



 下载:
下载:








 粤公网安备 44010502001741号
粤公网安备 44010502001741号
